The influence of transformers, induction motors and fault resistance regarding propagation voltage sags

Propagación de Huecos de Tensión: La influencia de transformadores, motores de inducción y resistencias de falla en relación con la propagación de huecos de tensión

J. Blanco¹ , R. Leal² , J. Jacome² , J.F. Petit³ , G. Ordoñez⁴ and V. Barrera⁵

¹ Jairo Blanco received the B.Sc. degree in electrical engineering from the Universidad Industrial de Santander (UIS), Bucaramanga, Colombia, in 2009. Currently, he is pursuing master studies in electrical engineering at Universidad Industrial de Santander. Email: jablas_19@hotmail.com.

² Ruben Darío Leal and Jonathan Jacome received the B.Sc. degree in electrical engineering from the Universidad Industrial de Santander (UIS), Bucaramanga, Colombia, in 2010. They belonged to the Research Group on Electric Power Systems GISEL. Emails: chile198822@hotmail.com, jague87@hotmail.com.

³ Johann F. Petit received the B.Sc. and M.Sc. degrees in electrical engineering from the Universidad Industrial de Santander (UIS), Bucaramanga, Colombia, respectively. He received his PhD in Electrical Engineering from the Universidad Carlos III from Madrid (UC3M), Spain. Currently, he is Titular Professor of the School of Electrical Engineering at the Universidad Industrial de Santander (UIS-Colombia). E-mail: jfpetit@uis.edu.co.

⁴ Gabriel Ordoñez received the B.Sc. degree in electrical engineering from the Universidad Industrial de Santander (UIS), Bucaramanga, Colombia, in 1985. He received his PhD in Industrial Engineering from the Universidad Pontificia Comillas, Madrid, Spain, in 1993. Currently, he is Titular Professor of the School of Electrical Engineering at the Universidad Industrial de Santander (UIS-Colombia). E-mail: gaby@uis.edu.co.

⁵ Victor Barrera received the B.Sc. and M.Sc. degrees in electrical engineering from the Universidad Industrial de Santander (UIS), Bucaramanga, Colombia, in 2003 and 2006, respectively. He is currently doing his PhD studies in the University of Girona, Catalonia, Spain. Email: victor.barrera@udg.edu.

ABSTRACT

Keywords: Power quality, voltage sag propagation, voltage dip causes, transformer connection.

RESUMEN

Palabras Claves:: Calidad de la energía eléctrica, propagación de huecos de tensión, causas de huecos de tensión, conexión de transformadores.

1. Introduction

Electricity service quality is generally related to service continuity and voltage wave quality. The voltage supplied to a load or installation is characterised by five parameters: frequency, magnitude, waveform, imbalance and continuity. Supply quality can be defined in terms of parameters deviating from their ideal values and defining maximum deviations without affecting electrical equipment operation.

Increased nonlinear loads such as computers, variable speed drives, robotic equipment and rectifiers have caused a significant increase in electromagnetic disturbances in power systems (voltage sags, swells, voltage spikes, harmonics, etc.). The presence of such disturbances in power system causes a decrease in operating efficiency (Chapman, 2001; Baggini, 2008). The importance of studying voltage sags lies in generating large losses in the industry and the economic sanctions being applied to electricity utilities by regulators.

Voltage sags have thus become more relevant during recent years because RMS voltage decreases and increases can cause malfunction or even total failure of electrical equipment operation (Bollen, 2000).

Due to the new electricity market and an increase in problems related to power quality (PQ), utilities have programmes for monitoring networks and identifying PQ disturbances. This article was thus aimed at reviewing PQ studies, specifically in the area of voltage sags, their causes and their propagation through a distribution network involving motors, transformers and capacitor banks.

ATPDraw was used for simulations in the IEEE 34 node test feeder and Matlab for data processing. The influence of fault resistance on voltage sag propagation was analysed and their considerations compared to some analysis presented in previous works (Aung and Milanovic, 2006; Mendes et al., 2008). The study of phenomena causing electromagnetic disturbances in power systems provides the information needed to improve the quality of power distributed to end-users.

2. Basic concepts related to voltage SAG propagation

According to IEEE standard 1159 (2009), voltage sags are defined as decreased rms voltage between 0.1 and 0.9 p.u to network frequency, lasting between 0.5 cycles and 1 minute. PQ disturbance magnitude and duration thus appear as main features. The literature related to voltage sags also identifies other features such as phase-angle jumps, starting points and recovery and wave distortion. However, it should be noted that voltage magnitude and phase angle jump characteristics are fundamental in analysing the propagation of such disturbances (Cornick and Li, 2000; Bollen and Zhang, 2000; Bollen and Zhang, 1999; Castellanos and Carrillo, 2003). The changes which voltage sag is subject to while it spreads throughout an electrical system are estimated from these characteristics.

The causes of this type of PQ disturbance are largely electrical faults and other events such as starting large induction motors, energising transformers and load increases (Bollen et al., 2007). It is thus interesting to analyse the effects on voltage sag propagation caused by this set of causes.

Voltage sags have been classified into three-phase systems by diagramming phase voltages according to fault type. Bollen (2000) presented a voltage sag classification with their respective equations defining the voltages for each type of sag and phasor diagram. This classification has been adopted and implemented in a variety of methodologies for characterising voltage sags and taken into account when analysing the following sections (Bollen and Zhang, 1999).

Bollen (2000) also discussed the influence of overhead lines and underground cables on voltage sag propagation, using the voltage divider model and considering sag magnitude as a function of the distance to the fault.

Figure 1 shows sag magnitude as a function of the distance to the fault for an 11 kV overhead line. The fault levels selected were 750 MVA, 200 MVA and 75 MVA (Bollen, 2000).

According to Figure 1, voltage sag magnitude increased (less severe sag) with increasing distance to a fault and fault level. It can also be seen that a fault 10 km away would produce a more severe sag. The voltage sag was less severe on the low-voltage side of a transformer due to the reflected impedance of high voltage level to low voltage level. The cross-section of overhead lines and underground cables' conductors would affect voltage sag propagation. Increased electrical conductor cross-section would produce severer sag magnitude for the same short-circuit level and operating voltage. This is because the reactive part of overhead lines is about 3 times larger than in underground cables and the resistive component tends to decrease with increasing cross-section.

3. Influence of transformer winding connections on voltage SAG propagation

Transformer winding connections used in transmission and distribution systems are analysed to estimate the influence of transformers on voltage sag propagation, of one voltage level to another.

Three-phase transformers can be classified into three types regarding voltage sag propagation between a transformer's primary and secondary sides (Aung and Milanovic, 2006; Mendes et al., 2008; Guasch and Córcoles, 2006). Table 1 shows that the type of sag was subjected to starting load and grounded neutral. The load was connected to the transformer's secondary side when such connection to the transformer's primary side would produce seven types of sag (Mendes et al., 2008).

The circuit shown in Figure 2 was modelled in ATPDraw to reproduce some of these cases shown in Table 1. It was a basic electrical system having a voltage sag generator. The voltage sags were obtained at the common connection point P_CC1, corresponding to the transformer's primary side. Some of those results are shown in Table 2.

Table 2 shows that when sags were propagated through the electrical system then their characteristics became altered due to the impedance between the event's source and load. The type of transformer winding connection could influence voltage sag propagation by modifying the magnitude and phase of the voltages in the power system buses. These results demonstrated that voltage sags' characteristics regarding the buses might be very different from those generated at the point of fault.

4. Induction motors influence on voltage SAG propagation

The voltage magnitude at an induction motor's terminals decreases when a three-phase fault occurs in an electrical system, thereby causing serious consequences (Castellanos and Carrillo, 2003; Yalçinkaya et al., 1998). The first refers to the imbalance between the air gap flux and stator voltage; the flux decays with a time constant of up to several cycles and the induction motor contributes to the fault thereby taking more current.

Voltage sag causes a reduction in a machine's electrical torque while load torque remains constant, so that a machine rotates more slowly. During such deceleration, an induction motor draws more current having a lower power factor, thereby increasing voltage drop (Guasch and Córcoles, 2006; Yalçinkaya et al., 1998). An important aspect is that a new steady state at a slower rate can be reached for small voltage drops, depending on resistant torque's mechanical load. If there are large voltage drops then a motor coasts to a stop or until the voltage reaches its nominal value again. Because the mechanical time constant is about one second or more, an induction motor usually does not reach zero speed.

The opposite happens when voltage recovers. The magnetic flux in the gap increases again and causes a current that slows recovery voltage. Subsequently, the motor accelerates to pre-fault speed, again taking a large current with low power factor and causing post-fault voltage sag having significant duration.

Moreover, the behaviour of an induction motor with unbalanced fault is more complex, to the point that the effects generated during this event can only be quantified by network analysis programmes (Guasch and Córcoles, 2006; Yalçinkaya et al., 1998). The interaction between the system and the induction motor during an unbalanced fault is initially characterised by a motor's contribution to the fault around the first two cycles. This causes an increase in positive sequence voltage while negative and zero sequence voltages are not influenced. Motor speed decreases, causing a drop in positive sequence impedance and the current increases consecutively while positive sequence voltage decreases. Since a motor's negative sequence impedance is low (between 10% and 20% of positive sequence impedance), negative sequence voltage is mitigated in the motor terminals. The induction motor does not take a zero sequence current, therefore, zero sequence voltage is not influenced by the engine.

5. Case study

This article presents the results of simulating the propagation of voltage sags obtained from modelling IEEE 34 node test feeder (2000) in ATPDraw. Analysing voltage sag propagation due to network faults and starting induction motors, energising transformers and network failures with fault resistance is this work's main contribution to propagation studies.

A. Modelling the electrical system

IEEE 34 node test feeder consists of 34 nodes, voltage being rated 24.9 kV at 60 Hz. It is characterised by very long light loads, unbalanced loading with both ''spot'' and ''distributed'' loads and shunt capacitors. The diagram for the system is shown in Figure 3.

Some modifications were made to the original electrical system when searching for some variables of interest in the study. The first was including an induction motor and its respective transformer. This induction motor was added to node 890 to obtain voltage sag by starting this engine. The induction motor was selected from Yalçinkaya et al., (1998). Its parameters are shown in Table 3. A transformer supplying a motor at rated voltage was placed between nodes 832 and 888.

The second change was inserting a transformer between nodes 836-862 which had a magnetisation curve for the core saturation effect. This produced voltage dips caused by energising transformers. Transformer parameters are shown in Table 4.

The graphs presented in the following sections are in p.u regarding 20.4 kV (high voltage side) and 3.4 kV base voltage (low-voltage side).

B. Propagating voltage sags caused by transformer energization

The voltage sags for this case were generated by energising the transformer connected between nodes 836-862. The difference in transformer energising with or without load was voltage sag magnitude, being less severe for the case without load (Bollen et al., 2007; Smith et al., 1999; Ahn et al., 2004). Figure 4 presents the rms voltage and current at node 836 (primary transformer)

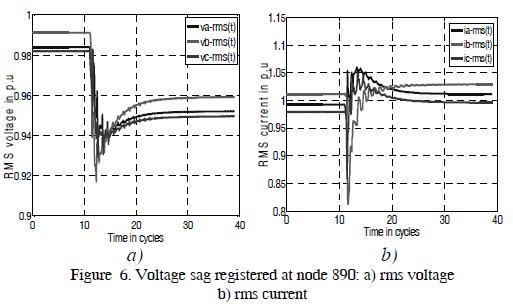
Although the peak current resulting in energising the transformer was 4.33 p.u, effective current only reached 2.15 p.u and voltage sag magnitude was around 0.85 p.u. Figure 5 shows that voltage sag had a waveform similar to that recorded at node 836, but as it spread to the substation (node 800) the depth decreased, being about 0.95 p.u, representing no voltage sag. Voltage dip phasor diagrams at node 862 (Dy1 transformer secondary) and node 890 (Yy transformer secondary) corresponding to induction motor terminals was also examined.

According to previous results, the voltage sag caused by transformer energising did not suffer significant effects due to connecting the transformers being tested (Yy and Dyn). Figure 6.d shows that the voltage sag had an angle jump due to connecting the Dyn transformer windings, but that only meant a rotation of the phasors without affecting sag magnitude or angular difference. Induction motor terminal voltage behaviour during voltage sag caused by transformer energising is shown in Figure 6.

An induction motor takes more current during voltage sag trying to keep its speed due to the voltage reduction in their terminals. As the voltage recovers, the current drawn by the induction motor increases rapidly, even attempting to overcome its pre-event current value, finally reaching steady state operation (Figure 6. B). Reducing rms current confirmed that the disturbance originated upstream of the point of induction motor connection.

It should be noted that several loads on an electrical system would be subject to voltage sag, especially those near the point of transformer energising.

C. Propagation voltage sags due to the induction motor starting

In this case, the induction motor was started having a load equal to 75% rated load. By energising the motor (node 890), the starting current reached values 4 times their rated value, generating a 0.35 p.u voltage sag. Figure 7 shows the phasor d iagram for voltage events at different points of interest.

The motor drew a large current to generate voltage drops throughout the circuit. The disturbance was not considered voltage sag at the substation node due to the recovery of sag magnitude. Figure 7 d. shows the characteristics of voltage sag after being transferred by two transformers (Yy and Dyn). The voltage sag recovered when it was transferred through transformer Yy. It also had a 30 degree lag inserted by the type 3 transformer.

D. Propagation of voltage sags due to network faults

This section discusses voltage sag propagation due to external electrical system faults. Some nodes were selected to show interesting results.

The first case concerned a single line-to-ground fault at node 862 (transformer secondary-type 3).

Figure 8 shows that the voltage sag was subject to important effects during its propagation. It was transferred through the transformer-type 3 as a two-phase voltage sag (phases a-b). At node 800, the voltages recorded were 0.879 p.u, 1 p.u and 0.932 for phases a, b and c respectively. This indicated that voltage dip was still considered despite recovery in sag magnitude during propagation. Similarly, groups connecting the transformers had no influence since they only involved a 120° rotation between primary and secondary voltages. Capacitor banks installed between 834-848 nodes generated some transients due to voltage changes but only having short duration (Hur and Santoso, 2008).

The results obtained after analysing voltage sag propagation caused by different types of faults at different points in a power system are summarised below.

Changes in the magnitude and phase of the voltage sag to be transferred through the transformer-type 3 (Dyn) occurred regarding line-to-line fault; these changes were shown in faulted and non-faulted phases. The transfer of voltage sag through the transformer-type 2 (Yy) did not alter its shape due to the absence of a zero-sequence voltage component.

Table 6 and 7 show some results demonstrating the effect of an induction motor on voltage sag due to an unbalanced fault, since the induction motor took more current, causing more severe voltage sag at its terminals.

E. Influence of fault resistance on voltage sag transfer

Fault resistance may change the type of voltage dip in relation to its magnitude or phase angle. Such resistance is mainly due to high and medium voltage support lines, the power system grounding circuit and soil conditions (García, 2010).

i. Influence of ground fault resistance (R_F)

A single line-to-ground fault (phase a) was generated at node 834 to analyse the influence of ground fault resistance Rf, and a record was taken of voltage magnitude and phase angles as a function of ground fault resistance. The results are shown below.

As shown in Figure 9, voltage sag caused by single line-to-ground fault at node 834 was transferred to the type 3 transformer's low-voltage side (node 862) as a line-to-line fault. This record was characterised by a reduction in phase b voltage magnitude with increasing fault resistance (up to about 16 ohms) and consecutively the voltage dip became less severe. Figure 10 shows induction motor influence, making voltage sag more severe.

ii. Influence of line-to-line fault resistance (R_FF)

Voltage sags caused by line-to-line faults have important differences in their magnitudes. Ideally, faulted phase voltage magnitudes should be equal.

In this case, a line-to-line fault (b-c phases) was generated with resistance fault at node 834 and the results are presented below.

Figure 11 and Figure 12 show that by increasing the value of R_FF, there was a difference between the phases involved in the two-phase short circuit, which ideally should have provided similar magnitudes. Again, a critical resistance value was identified for which phase voltage changed its behaviour. The phase angle tended to change regarding pre-fault value as fault resistance decreased.

6. Conclusions

This article has presented an analysis of voltage sag propagation. It took into account the influence of the transformers winding connections, induction motors and fault resistance. A first important aspect referred to when the disturbance had a zero-sequence voltage component and the connection of the transformer windings blocked the flow of this sequence component. These effects were presented by type 2 and 3 transformers. In the case of voltage sags without zero-sequence component, the influence was only due to phase changes imposed by the type 3 transformer.

An analysis based on factors such as the cross section of lines and cables, characteristic impedance transformers, the distance to the fault and short circuit levels would lead to estimating voltage sag magnitude and phase characteristics at different points in an electrical system.

From the analysis of the propagation of voltage sags caused by induction motor starting and transformer energising it was concluded that these types of sag were only influenced by type 3 transformers, due to the absence of zero sequence voltage. This information is useful for characterising this type of voltage events at each node of interest.

Voltage dips can be modified regarding their magnitude and phase when a fault is generated through fault resistance. It was observed that there were some critical fault resistance values for which there was a change in voltage sag behaviour.

This is an important factor to consider in characterising unbalanced voltage dips due to the characteristics of the sags being associated to system elements when the effects of fault resistances are known.

ATPDraw was helpful in identifying these different effects on voltage sags and is presented as a tool having great potential for future work related to PQ disturbance propagation in power systems with the presence of other elements and loads such as variable speed drives, rectifiers, large rotating loads, reactors and voltage regulators.

7. References

Ahn S., Won D., Chung Y. and Moon S., Determination of the Relative Location of Voltage Sag Source According to Event Cause, IEEE, Power Engineering Society G.M, vol.1, June 2004, pp. 620-625.        [ Links ]

Aung M. and Milanovic J., The Influence of Transformer Winding Connections on the Propagation of Voltage Sags, IEEE Transactions on Power Delivery, Vol. 21, No. 1, January 2006, pp. 262-269.        [ Links ]

Baggini A., Handbook of Power Quality, Edition I, Jhon Wiley and Sons, Ltd, Dic. 2008, Chapter 4 and 18, pp. 79-131 and 556-558.        [ Links ]

Bollen M., Understanding Power Quality Problems. Voltage Sag and Interruptions. IEEE PRESS. New York. 2000.        [ Links ]

Bollen M. and Zhang L., A Method for Characterisation of Three-Phase Unbalanced Dips from Recorded Voltage Waveshapes, IEEE Telecommunications Energy Conference, Chalmers of Electric of Technology, 1999. pp. 1-9.        [ Links ]

Bollen M. and Zhang L., Characteristic of Voltage Dips (Sags) in Power System, IEEE Trans. Power Del., Vol. 15, no. 2, Apr. 2000, pp. 827-832.        [ Links ]

Bollen M., Yu-Hua I., Axelberg P., and Styvaktakis E., Classification of Underlying Causes of Power Quality Disturbances: Deterministic versus Statistical Methods, EURASIP Journal on Advances in Signal Processing, Volume 2007, Article ID 79747, 17 pages.        [ Links ]

Castellanos C. and Carrillo L., Characterization of Voltage Sags, Degree thesis in Electrical Engeneering, Dir. Gabriel Ordoñez, Industrial University of Santander. 2003.        [ Links ]

Chapman D., The Cost of Poor Power Quality, Power Quality Aplication Guide, Cooper Development Association. March 2001, pp. 1-4.        [ Links ]

Cornick K. and Li H., Power Quality and Voltage Dips, IEEE 5th International Conference on Advances in Power System Control, Operation and Management, APSCOM 2000, Hong Kong, October 2000, Development Department Singapore Power Systems Lt, Vol. 1, pp. 149-156.        [ Links ]

García E., Assessment of Power Quality respect the voltage sags: Indices and quality levels, Degree thesis in engineering directed by Dr. Fuster Roig Vicente, Universidad Politecnica de Valencia.        [ Links ]

Guasch L. and Córcoles F., Effects of voltage sags on induction machines and three-phase transformers, Doctoral thesis, Universidad de Catalunya, Electrical Engineering department, Barcelona, January 2006, 138 pages.        [ Links ]

Hur K. and Santoso S., On Two Fundamentals Signatures for Determining the relative location of Switched Capacitor Banks, IEEE Transactions on Power Delivery, Vol. 23, No. 2, April 2008, pp. 1105-1112.        [ Links ]

IEEE 1159-1995, Recommended Practice of Monitoring Electric Power Quality, 1995.        [ Links ]

Mendes W., Samesima M. and Moura F., Influence of Power Transformer Winding Connections on the Propagation of Voltage Sags through Electric System, IEEE.. Electricity Market, 2008, EEM 2008, 5th International Conference on European, pp. 1-6.        [ Links ]

Radial Test Feeders- IEEE Distribution System Analysis Subcommittee. http://ewh.ieee.org/soc/pes/dsacom/testfeeders.html. Consulted January 2010.        [ Links ]

Smith K., Ran L. and Leyman B., Analysis of Transformer Inrush Transients in offshore Electrical Systems, IEEE Proc-Gener. Transm. Distrib., Vol. 146, No. 1,January 1999, pp. 89-95.        [ Links ]

Yalçinkaya G., Bollen M. and Crossley P., Characterization of Voltage Sags in Industrial Distribution Systems, IEEE Transactions on Industry Applications, Vo1.34, no.4, July 1998, pp.682-688.        [ Links ]