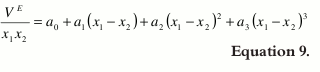INDUSTRIAL FARMACÉUTICA

Although the cosolvent system 1,4-dioxane + water is poisonous, it is widely used in the pharmaceutical sciences as a versatile model for studying drug solubility and some other relevant physicochemical properties in the design of homogeneous dosage forms. For this reason, the excess molar volumes and partial molar volumes of components are investigated from density measurements of the entire range of mass fractions for the 1,4-dioxane + water system at 293.15 K; 298.15 K; 303.15 K; 308.15 K and 313.15 K. The results of excess molar volumes are fitted by Redlich-Kister equation using third degree polynomials and compared with those of literature for other systems. The system exhibits large negative excess volumes (up to - 0.71 cm³ mol^-1) probably due to increased interactions like hydrogen bonding between unlike molecules or very large differences in the molar volumes of pure components. The effect of temperature on the different volumetric properties studied is also analyzed. Besides, the volume thermal expansion coefficients are also calculated founding values varying from 2.50 x 10^-4 K^-1 for pure water up to 1.06 x 10^-3 K^-1 for pure 1,4-dioxane at 298.15 K.

Keywords: 1,4-dioxane, water, binary liquid mixtures, excess volumes, partial volumes.

Aunque el sistema cosolvente 1,4-dioxano + agua es tóxico, este ha sido ampliamente utilizado en las ciencias farmacéuticas como un modelo versátil en el estudio de la solubilidad de fármacos y de otras propiedades fisicoquímicas de interés en el diseño de formulaciones líquidas homogéneas puesto que cubre un amplio espectro de polaridades. Por esta razón, en este trabajo se calculan los volúmenes molares de exceso y molares parciales a partir de medidas de densidad en el sistema 1,4-dioxano + agua en todo el intervalo de composición a 293,15 K; 298,15 K; 303,15 K; 308,15 K y 313,15 K. Los resultados de volumen molar de exceso son modelados de acuerdo a la ecuación de Redlich-Kister usando polinomios regulares de tercer grado y comparados con otros presentados en la literatura para otros sistemas. El sistema estudiado presenta volúmenes de exceso altamente negativos (hasta - 0,71 cm³ mol^-1) probablemente debido a las fuertes interacciones por unión de hidrógeno entre las moléculas de los dos compuestos y a la gran diferencia en los volúmenes molares de los dos componentes puros. También se analiza el efecto de la temperatura sobre las diferentes propiedades volumétricas estudiadas. Así mismo se calculan los coeficientes térmicos de expansión volumétrica encontrado valores desde 2,50 x 10^-4 K^-1 para el agua pura hasta 1,06 x 10^-3 K^-1 para el 1,4-dioxano puro a 298,15 K.

Palabras clave:1,4-dioxano, agua, mezclas líquidas binarias, volúmenes de exceso, volúmenes parciales.

Water-cosolvent mixtures have been used widely in pharmacy in order to increase the solubility of drugs that are poorly soluble in water during the design of homogeneous pharmaceutical dosage forms, such as syrups and elixirs, among others (1, 2). 1,2-propanediol and ethanol are the cosolvents most used in design nowadays, especially those that are intended for elaboration of peroral and parenteral medications (3). Several examples of pharmaceutical formulations using these cosolvents have been presented by Rubino (1). Nevertheless, 1,2-propanediol and ethanol are hydrogen-donor and hydrogen-acceptor solvents, and they have relatively large dielectric constants (24 and 32 at 293.15 K (4)) and thus, mixtures with low polarities could not be studied by using these two solvents and water. Otherwise, 1,4-dioxane is a toxic organic solvent, miscible with water in all possible compositions, although it has a low dielectric constant (2.2 at 293.15 K (4)). For this reason, this solvent allows to study polarities from 2 to 80 at room temperature when is blended with water. On the other hand, 1,4-dioxane acts only as a Lewis base in aqueous media, opposite to 1,2-propanediol and ethanol, which act as donor and acceptor as well. Although this is a toxic solvent, it has been widely used as model cosolvent for drugs' solubility studies developed by several authors (5-8).

The cosolvent mixtures obtained using these cosolvents and water are highly non-ideal due to increased interactions between unlike molecules and large differences in molar volumes of pure components, which leads to non-additive volumes on mixing (9, 10). For this reason it is necessary to characterize the volumetric behavior of these binary mixtures as a function of temperature in order to extend the physicochemical information available for liquid mixtures used in pharmacy, including those with poisonous behavior. This information is useful for predicting the intermolecular interactions present in liquid pharmaceutical systems (11).

In this report, the excess molar volumes and the partial molar volumes of the binary system of 1,4-dioxane + water at various temperatures in addition to other volumetric properties were calculated according to modified procedures widely exposed in literature (12-15). This report is a continuation of those presented previously about some volumetric properties of ethanol + water (16) and 1,2-propanediol + water mixtures (17). As was already said, 1,4-dioxane has only proton-acceptor groups, which imply a significant degree of H-bonding with water, leading to mutual association in their binary mixtures (10).

Materials

In this investigation, dehydrated 1,4-dioxane from Scharlau, Spain, and distilled water (conductivity < 2 mS cm^-1) were used. The dehydrated 1,4-dioxane employed was maintained over molecular sieve to obtain a dry solvent previously to prepare the cosolvent mixtures. The dryness obtained for 1,4-dioxane was demonstrated by the density value obtained which was coincident with those presented in literature (18, 19). Prior to mixtures preparation, distilled water was treated in an Elmasonic E 60 H ultrasonic bath at room temperature for 10 min. to remove dissolved air. Cosolvent mixtures preparation

All 1,4-dioxane + water cosolvent mixtures were prepared in quantities of 40.00 g by mass using a Ohaus Pioneer TM PA214 analytical balance with sensitivity ± 0.1 mg, in concentrations from 10% m/m to 90% m/m varying in 10% m/m, to study nine mixtures and the two pure solvents. This procedure imply an uncertainty of ± 2 x 10 ^-5 in mole fraction. The mixtures were allowed to stand in Magni Whirl Blue M water baths at temperatures from 293.15 K to 313.15 K varying in 5.00 ± 0.05 K for at least 30 minutes previous to density determinations.

Density determination

This property was determined using a DMA 45 Anton Paar digital density meter connected to a Neslab RTE 10 Digital Plus (Thermo Electron Company) recirculating thermostatic water bath according to a procedure previously described (20). The equipment was calibrated according to Instruction Manual using air and water at the different temperatures studied (21). All mixture preparations and density measurements were repeated at least five times and the results were averaged. From density values all thermodynamic properties were calculated as will be indicated in the next section.

Table 1 presents the composition of 1,4-dioxane + water mixtures, in mass percent and mole fraction, in addition to density values at several temperatures studied. Our density values are in agreement with those presented by Morcom and Smith (22) at 298.15 K and also with those presented by Matsuo et al (23). The differences found among all literature data were in general lower than 0.0003 g cm^-3. Nevertheless, some differences have been found with respect those presented by Nayak et al (24) at 303.15 K and 313.15 K, in particular, in 1,4-dioxane-rich mixtures. In all cases, the density decreases almost linearly as the temperature increases except for water.

Table 1. Densities^{(a, b)} for 1,4-dioxane + water mixtures at various temperatures.

Molar volumes and excess molar volumes

In table 2 the molar volumes for binary mixtures at all temperatures are presented which were calculated from equation 1.

where, x₁ and x₂ are the mole fractions and M₁ and M₂ are the molar masses, for both components respectively, and ρ is the mixture density.

Table 2. Molar volumes, excess molar volumes. and partial molar volumes of components for 1,4-dioxane + water mixtures at various temperatures.

On the other hand, the excess volumes calculated from equation 2 (where, ρ₁ and ρ₂ are the densities of pure components) at all temperatures studied are also presented in table 2. This behavior is shown graphically in figure 1 at 298.15 K.

Figure 1. Excess molar volumes of 1,4-dioxane + water mixtures at 298.15 K.

Analogous to the behavior obtained in other investigations (10, 16, 17), in all cases the excess volumes are largely negative (especially around 0.32 in mole fraction of 1,4-dioxane, where it is near to 0.71 cm³ mol^-1) indicating contraction in volume. As was already said (16, 17), according to Fort and Moore (25), a negative excess volume is an indication of strong heteromolecular interactions in the liquid mixtures and is attributed to charge transfer, dipole-dipole, dipole-induced dipole interactions, and hydrogen bonding between the unlike components, while a positive sign indicates a weak interaction and is attributed to dispersion forces (London interactions) which are likely to be operative in all cases.

In the evaluated system, where the hydrogen bonding predominates, the contraction in volume has been interpreted basically in qualitative terms considering the following events, first: expansion due to depolymerization of water by addition of 1,4-dioxane; second: contraction due to free volume difference of unlike molecules; and third: contraction due to hydrogen bond formation between 1,4-dioxane and water through -OH---O< bonding (10).

Thus, the large negative values of V^E over the free volume contribution indicate the presence of strong specific interactions with predominance of formation of hydrogen bonds between 1,4-dioxane and water over the rupture of hydrogen bonding in water-water.

The excess molar volumes becomes less negative as the temperature is raised indicating volume expansion which indicates the decrease in the interactions between 1,4-dioxane and water molecules with increase in temperature.

Partial molar volumes

The partial molar volumes for 1,4-dioxane () and water () were calculated using the classical Bakhuis-Roozeboom method by means equations 3 and 4 applied to the variation of the respective specific volumes as a function of 1,4-dioxane mass fraction (table 2) and adjusting them to third degree polynomials by least squares regression analyses (11, 26, 27). Polynomials of third degree order are the most widely used in these cases, based upon their good statistical parameters, specially their determination coefficients (figure 2). The first derivatives were taken out on the polynomials obtained and solved at each composition point.

Figure 2. Specific volume as a function of the mixtures composition. 293.15 K (○), 298.15 (□), 303.15 K (△), 308.15 (◇), 313.15 ( ×).

The and values are also presented in table 2, in addition to the slopes obtained (dV/dµ_D) at each composition and temperature. Almost in all cases the partial molar volumes for 1,4-dioxane and water in the mixtures are lower than those for the pure solvents (except for water in mixtures with 10% m/m of 1,4-dioxane at 293.15 K and 298.15 K). The partial volumes for 1,4-dioxane varied from 80.39 cm³ mol^-1 (for 10% m/m of 1,4-dioxane at 293.15 K) to 87.15 cm³ mol^-1 (for 90% m/m of 1,4-dioxane at 313.15 K), and for water varied from 18.17 cm³ mol^-1 (for 10% m/m of 1,4-dioxane at 313.15 K) to 16.85 cm³ mol^-1 (for 90% m/m of 1,4-dioxane at 293.15 K). Our results for are almost coincident with those presented at 298.15 K by Matsuo et al (23). The results obtained for and are in agreement with the negative excess volumes obtained. The variation of this property is presented in Figure 3 as a function of 1,4-dioxane mole fraction at 298.15 K for 1,4-dioxane and water respectively. These values were calculated as the difference between partial molar volumes and molar volumes presented in table 2. For both solvents the partial molar volume diminishes as their respective proportion in the mixtures diminishes.

Figure 3. Partial molar volumes of water (circles) and 1,4-dioxane (squares) calculated as differences respect to pure solvent volumes in 1,4-dioxane + water mixtures at 298.15 K.

At this point it is important to consider that partial volumes were obtained by using specific volumes and mass fractions, in opposite way to that done previously with ethanol + water mixtures (16) and 1,2-propanediol + water mixtures (17). For 1,4-dioxane + water system straight lines were obtained for molar volume vs. mole fraction, which apparently indicates no variation of partial molar volumes with respect to molar volumes as pure compounds. Figure 4 shows the lineal behavior obtained at 298.15 K. For this reason, the classical method based on molar volumes and mole fractions was not used in this case.

Figure 4. Molar volume as a function of the mixtures composition expressed as mole fraction at 298.15 K.

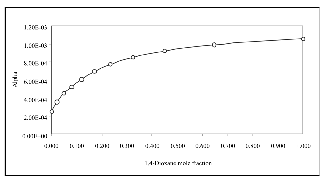
On the other hand, a method based on the variation of apparent molar volume (Φ_V) of 1,4-dioxane with respect to cosolvent composition was also developed to obtain the partial molar volumes. Accordingly to equation 5, Φ_V is defined as:

where V is the mixture molar volume, V_W⁰ is the molar volume of water as pure compound (18.07 cm³ mol^-1 at 298.15 K), and xD and xW are the mole fractions of 1,4-dioxane and water, res­pectively. Table 3 and figure 5 shows the behavior of Φ_V with respect to cosolvent composition at 298.15 K. It is clear that Φ_V increases as the 1,4-dioxane proportion increases in the mixtures. This behavior has been associated to water-structure breaking effects due to dissolved solutes (28).

Table 3. Apparent molar volumes and partial molar volumes of components for 1,4-dioxane + water obtained from the variation of apparent molar volumes with respect to 1,4-dioxane moles.

Figure 5. Apparent molar volume of 1,4-dioxane in water at 298.15 K.

The variation of Φ_V with respect to x_D was adjusted to a regular polynomial of third degree. was calculated according to:

where Φ_V⁰ is the apparent molar volume of 1,4-dioxane in water at infinite dilution (it is coincident with at concentration 0 of 1,4-dioxane) and n₂ is the number of 1,2-dioxane moles. The values obtained at 298.15 K are also presented in Table 3. increases as the 1,4-dioxane proportion increases in the mixtures. On similar way, was calculated according to equation 7:

The values as a function of cosolvent composition are also presented in table 3. diminishes as the water proportion is reduced in the mixtures.

By comparing the partial molar volumes obtained by both methods it could be stated that the trends obtained are similar, but the quantitative results are slightly different, in particular at large proportion of each component in the mixture.

Redlich-Kister equation

As was already said (16, 17), Redlich and Kister (29) introduced in 1948 the general form of equation 8 to facilitate the representation of thermodynamic properties and the classification of solutions in multicomponent systems, especially those important in petroleum chemistry. The Redlich-Kister Equation has been used for various decades for manipulating several kinds of physicochemical values of mixtures such as: excess volumes, excess viscosities, solubilities in cosolvent mixtures, among others.

In the analysis of our data about excess volumes the equation 8 was used in the form of third degree polynomial equations using least square analyses, and therefore, obtaining four coefficients as presented in equation 9. Polynomials of third degree are the most widely used in this case again, because of their relevant statistic parameters such as determination coefficients and standard deviations.

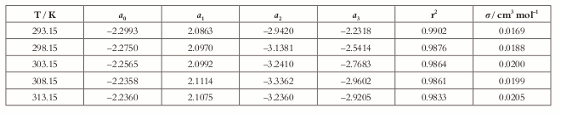
The Redlich-Kister parameters for 1,4-dioxane + water mixtures at all temperatures studied are presented in table 4 in addition to determination coefficients and standard deviations calculated according to equation 10 (where D is the number of compositions studied and N is the number of terms used in the regression, that is 9 and 4 respectively in our case). Equation 10 has been widely used in literature (16, 17). Figure 6 shows the Redlich-Kister equation applied to 1,4-dioxane + water data at 298.15 K.

Table 4. Redlich-Kister regression results for the excess volumes of 1,4-dioxane + water mixtures at various temperatures in mole fraction.

Figure 6. Regression adjusted to Redlich-Kister equation using four terms for 1,4-dioxane + water mixtures in mole fraction at 298.15 K.

The variation coefficients are greater than 0.983 indicating that the obtained regular polynomials regressions describe adequately the excess volumes, because the standard deviations are similar to those presented in literature for other mixtures (12-15). On the other hand, σ values obtained for 1,4-dioxane + water mixtures were in general lower than those obtained for ethanol + water (16) and 1,2-propanediol + water (17) mixtures when third degree regular polynomials were used as well.

Volume thermal expansion

On the other hand, in pharmaceutical and chemical preformulation studies is very important to predict the variation of physicochemical properties related to pharmaceutical dosage forms, with respect to temperature changes; specially those properties which affect the concentration of active ingredients in the developed formulations. For this reason the volume thermal expansion coefficients (α) were calculated by means of equation 11 (30) by using the variation of molar volumes with temperature (table 2).

Table 5 summarizes the (δV/δT) and α values for all mixtures and pure solvents whereas Figure 7 shows the volume thermal expansion coefficients at 298.15 K. For all mixtures and pure solvents, quadratic polynomial models were used, obtaining determination coefficients greater than 0.999. The a values varied from 2.50 x 10^-4 K^-1 in water up to 1.06 x 10^-3 K^-1 in pure 1,4-dioxane. From 0 to 0.3 in mole fraction of 1,4-dioxane the a values increase readily. In a first approach this fact would be explained in terms of water-structure loosing by addition of 1,4-dioxane. It should be kept in mind that over 0.3 in mole fraction of 1,4-dioxane the most contributing component to all mixture volume is 1,4-dioxane, which is also the less polar solvent in these mixtures (1, 2).

Table 5. Volume thermal expansion coefficients of 1,4-dioxane + water mixtures at 298.15 K.

Figure 7. Volume thermal expansion coefficients (K^-1) for 1,4-dioxane + water mixtures at 298.15 K.

Variation of excess molar volume with temperature

An additional and important treatment is the evaluation of change of the excess molar volumes with temperature (δV^E/δT). Figure 8 shows this property at 298.15 K (this value is constant over the entire temperature interval considered, that is, from 293.15 K to 313.15 K), which was obtained considering linear behavior of (δV^E/δT) in all mixtures studied (30). In almost all cases the determination coefficient values obtained were greater than 0,97. From figure 8 it follows that there is only a tendency according to composition, that is, this property is always positive, which reflects the fact that excess volume decreases with increasing temperature. This result could be due to weakening of hydrogen-bonding as the temperature increases which could lead to solvent structure loosing, and thus, leading to more ideal mixing behavior.

Figure 8. Change of the excess molar volumes with temperature (cm³ mol^-1 K^-1) for 1,4-dioxane + water mixtures at 298.15 K (From 293.15 to 313.15 K).

Variation of excess molar enthalpy with pressure

Finally, from the excess molar volumes presented in table 2 the change of the excess molar enthalpies with pressure according to equation 12 (30), was calculated:

Figure 9 shows (δH^E/δp) values at 298.15 K where it follows that this property is negative in all compositions, indicating an increase in the excess molar enthalpy as the pressure is increased. Unfortunately, there is not available experimental data in literature about this property for our system. Although, Suzuki et al (31) made a calorimetric study on heat of mixing of 1,4-dioxane and water founding excess molar enthalpies negative from 0 to 0.4 in mole fraction of 1,4-dioxane and positive for the other mixtures at all the temperatures studied (from 298.15 K to 338.15 K).

Figure 9. Change of the excess molar enthalpies with pressure (J mol^-1 MPa^-1) obtained from the excess molar volumes for 1,4-dioxane + water mixtures at 298.15 K.

Accordingly to Suzuki et al (31), the exothermic behavior shows that new strong hydrogen bonds might be formed in the water-rich region probably due to hydrophobic hydration around ethyl moieties of 1,4-dioxane. On the other hand, the same authors propose three composition regions based on the variation of partial molar enthalpies of water and 1,4-dioxane. These regions are characterized according to the aggregate species present as clusters in each of them. On the other hand, according to Takamuku et al (32), large hydrogen bonded clusters corresponding to water are dominant at xD < 0.1, whereas for 0.1 < x_D < 0.3 a structural transition is presented, where hydrogen bonded clusters of 1,4-dioxane and several free water molecules are formed.

On the other hand, some other experimental techniques have been used to evaluate the nanoscopic structure of these mixtures (32, 33). Among these techniques, X-ray diffraction (32) and mass spectrometry (32) have been used to evaluate static structural properties and NMR relaxation (32) and thermal effusivity (33) to evaluate dynamic properties. In general, these techniques corroborate that described by means of thermodynamic methods.

This report expands widely the experimental volumetric information about the 1,4-dioxane + water cosolvent system available nowadays (22-24) because it includes the behavior at five temperatures commonly found in technological conditions. As it was said earlier, this information could be employed in several engineering processes and for the theoretical understanding of the behavior of cosolvent mixtures used in the chemical and pharmaceutical industries. In general terms, based in our results and those presented in the literature for other experimental and theoretical procedures, it can be concluded that 1,4-dioxane + water mixtures clearly show non ideal behavior. Nevertheless, the observed deviations are lower than those observed earlier for aqueous mixtures containing ethanol and propylene glycol as cosolvents. These observations demonstrate clearly that it is necessary to characterize systematically this important binary system in order to have complete experimental information about the physical and chemical properties useful in the understanding of liquid pharmaceutical systems. It is important to keep in mind that the experimental information obtained for binary solvent mixtures could be employed to estimate the physicochemical behavior of ternary and quaternary mixtures by means of theoretical methods such as the one described by Mehlman et al (34) based on artificial neural networks, among others. Besides, several methods have been described in the literature to estimate activity coefficients from excess functions in binary mixtures (35). Ultimately, based on thermophysical properties of liquids Marcus (36) described some methods intended to the understanding the preferential solvation of drugs and other solutes in binary solvent mixtures.

We thank the DIB of the Universidad Nacional de Colombia (UNC) by the financial support in addition to the Department of Pharmacy of UNC for facilitating the equipment and laboratories used in this investigation.

1. Rubino JT. Cosolvents and cosolvency. In: Swarbrick J, Boylan JC, editors. Encyclopedia of Pharmaceutical Technology, Vol. 3. New York: Marcel Dekker; 1988. pp. 375-98.        [ Links ]

2. Yalkowsky SH. Solubility and solubilization in aqueous media. New York: American Chemical Society and Oxford University Press; 1999. pp. 180-235.        [ Links ]

3. Pérez DC, Guevara CC, Cárdenas CA, Pinzón JA, Barbosa HJ, Martínez F. Solubilidad y volúmenes de desplazamiento del acetaminofén en mezclas binarias formadas por agua, etanol y propilenoglicol a 25.0 °C. Rev Colomb Cienc Quím Farm. 2003; 32 (2): 116-136.        [ Links ]

4. Sinko PJ. Martin's Physical Pharmacy and Pharmaceutical Sciences. 5 ed. Philadelphia: Lippincott Williams & Wilkins; 2006.        [ Links ]

5. Martin A, Newburger J, Adjei A. Extended Hildebrand approach: solubility of caffeine in dioxane-water mixtures. J Pharm Sci. 1980; 69 (6): 659-661.        [ Links ]

6. Martin A, Wu PL. Extended Hildebrand solubility approach: p-Hydroxibenzoic acid in mixtures of dioxane and water. J Pharm Sci. 1981; 72 (6): 587-592.        [ Links ]

7. Bustamante P, Romero S, Peña A, Escalera B, Reillo A. Nonlinear enthalpy-entropy compensation for the solubility of drugs in solvent mixtures: paracetamol, acetanilide and nalidixic acid in dioxane-water. J Pharm Sci. 1998; 87 (12): 1590-1596.        [ Links ]

8. Jouyban A. In silico prediction of drug solubility in water-dioxane mixtures using the Jouyban-Acree model. Pharmazie. 2007; 62 (1): 46-50.        [ Links ]

9. Battino R. Volume changes on mixing for binary mixtures of liquids. Chem Rev. 1971; 71 (1): 5-45.        [ Links ]

10. Kapadi UR, Hundiwale DG, Patil NB, Lande MK, Patil PR. Studies of viscosity and excess molar volume of binary mixtures of propane-1,2 diol with water at various temperatures. Fluid Phase Equilibr. 2001; 192 (1): 63-70.        [ Links ]

11. Rodríguez S, Vargas RA, Pinzón JA, Barbosa HJ, Martínez F. Aplicación del método de Bakhuis-Rooseboom para el cálculo de volúmenes específicos parciales en sistemas ternarios. Rev Colomb Cienc Quím Farm. 2002; 31:11-18.        [ Links ]

12. Wahab MA, Azhar M, Mottaleb MA. Volumetric behaviour of binary liquid mixtures at a temperature of 303.15K. B Kor Chem Soc. 2002; 23 (7): 953-956.        [ Links ]

13. Salas JA, Zurita JL, Katz M. Excess molar volumes and excess viscosities of the 1-chlorobutane + pentane + dimethoxyethane ternary system at 298,15 K. J Argent Chem Soc. 2002; 90 (1-3): 61-73.        [ Links ]

14. Peralta RD, Infante R, Cortez G, Ramírez RR, Wisniak J. Densities and excess volumes of binary mixtures of 1,4-dioxane with either ethyl acrylate, or butyl acrylate, or methyl methacrylate, or styrene at T = 298.15 K. J Chem Thermodyn. 2003; 35 (2): 239-250.        [ Links ]

15. Resa JM, González C, Goenaga JM, Iglesias M. Temperature dependence of excess molar volumes of ethanol + water + ethyl acetate. J Solution Chem. 2004; 33 (2): 169-198.        [ Links ]

16. Jiménez J, Manrique J, Martínez F. Effect of temperature on some volumetric properties for ethanol + water mixtures. Rev Colomb Cienc Quím Farm. 2004; 33 (2): 145-155.        [ Links ]

17. Jiménez J, Martínez F. Study of some volumetric properties of 1,2-propanediol + water mixtures at several temperatures. Rev Colomb Cienc Quím Farm. 2005; 34 (1): 46-57.        [ Links ]

18. Budavari S, O'Neil MJ, Smith A, Heckelman PE, Obenchain Jr. JR, Gallipeau JAR, D'Arecea MA. The Merck Index, An Encyclopedia of Chemicals, Drugs, and Biologicals. 13 ed. Whitehouse Station (NJ): Merck & Co., Inc.; 2001.        [ Links ]

19. Lide DR. CRC Handbook of Chemistry and Physics. 84 ed. Boca Raton: CRC Press LLC; 2003.        [ Links ]

20. Martínez F, Gómez A, Ávila CM. Volúmenes molales parciales de transferencia de algunas sulfonamidas desde el agua hasta la mezcla agua-etanol (X = 0.5). Acta Farm Bonaerense. 2002; 21 (2): 107-118.        [ Links ]

21. Anton Paar KG. DMA 45: Calculating Digital Density Meter Instruction Manual. Graz, Austria; 1980.        [ Links ]

22. Morcom KW, Smith RW, Thermodynamic behaviour of aqueous solutions of cyclic ethers. Part 1. -Enthalpies and volumes of mixing. Trans Faraday Soc. 1970; 66: 1073-1080.        [ Links ]

23. Matsuo S, Mizuguchi Y, Tanaka Y, Kubota H, Yamamoto R. Volumetric properties of mixtures of 1,4-dioxane and water at high pressures. Int J Thermophys. 1996; 17 (2): 441-454.        [ Links ]

24. Nayak JN, Aralaguppi MI, Naidu BVK, Aminabhavi TM. Thermodynamic properties of water + tetrahydrofuran and water + 1,4-dioxane mixtures at (303.15, 313.15, and 323.15) K. J Chem Eng Data. 2004; 49 (3): 468-474.        [ Links ]

25. Fort RT, Moore WR. Viscosities of binary liquid mixtures. Trans Faraday Soc 1966; 62: 1112-1119.        [ Links ]

26. Kestin J. A Course in Thermodynamics. New York: McGraw- Hill; pp. 331-332.        [ Links ]

27. Torres DR, Blanco L, Martínez F, Vargas EF. Apparent molal volumes of lidocaine-HCl and procaine-HCl in aqueous solution as a function of temperature. J Chem Eng Data. 2007; 52 (5): 1700-1703.        [ Links ]

28. Perrot P. A to Z of Thermodynamics. New York: Oxford University Press, Inc.; 1998. pp. 221-225.        [ Links ]

29. Redlich O, Kister AT. Algebraic representation of thermodynamic properties and the classification of solutions. Ind Eng Chem. 1948; 40 (2): 345-348.        [ Links ]

30. Ott JB, Boerio-Goates J. Chemical Thermodynamics: Advanced Applications. London: Academic Press; 2000. pp 271-291.        [ Links ]

31. Suzuki T, Fujisawa M, Takagi S, Kimura T. Excess enthalpies of water + 1,4-dioxane at 278.15, 298.15, 318.15 and 338.15 K. J Therm Anal Calorim. 2006; 85 (3): 545-550.        [ Links ]

32. Takamuku T, Yamaguchi A, Tabata M, Nishi N, Yoshida K, Wakita H, Yamaguchi T. Structure and dynamics of 1,4-dioxane- water binary solutions studied by X-ray diffraction, mass spectrometry, and NMR relaxation. J Mol Liq. 1999; 83 (1-3): 163-177.        [ Links ]

33. Sikorska A, Lindea BB. Manifestation of hydration effects in dioxane-water solutions by concentration-dependent changes of thermal and elastic properties. Chem Phys. 2008; 354 (1-3): 148-154.        [ Links ]

34. Mehlman SA, Wentzell PD, McGuffin VL. Prediction of simple physical properties of mixed solvent systems by Artificial Neural Networks. Anal Chim Acta. 1998; 371 (2-3): 117-130.        [ Links ]

35. Prausnitz JM, Lichtenthaler RN, Gomes de Acevedo E. Molecular Thermodynamics of Fluid-Phase Equilibria. 2 ed. Englewood Cliffs, NJ: Prentice-Hall; 1986. pp. 181-186.\        [ Links ]

36. Marcus Y. Solvent Mixtures: Properties and Selective Solvation. New York: CRC Press; 2002. pp. 142-179.        [ Links ]

* Autor a quien debe dirigir la correspondencia: fmartinezr@unal.edu.co