Services on Demand
Journal
Article
Indicators
-
 Cited by SciELO
Cited by SciELO -
 Access statistics
Access statistics
Related links
-
 Cited by Google
Cited by Google -
 Similars in
SciELO
Similars in
SciELO -
 Similars in Google
Similars in Google
Share
CT&F - Ciencia, Tecnología y Futuro
Print version ISSN 0122-5383On-line version ISSN 2382-4581
C.T.F Cienc. Tecnol. Futuro vol.3 no.3 Bucaramanga Jan./Dec. 2007
A COMPLEMENTARY CONVENTIONAL ANALYSIS FOR CHANNELIZED RESERVOIRS
Análisis convencional complementario para yacimientos alagados
Freddy-Humberto Escobar1 and Matilde Montealegre-M.2
1,2Universidad Surcolombiana, Programa de Ingeniería de Petróleos, Grupo de Investigación en Pruebas de Pozos, Neiva, Huila, Colombia e-mail:fescobar@usco.edu.co, e-mail: matildemm@usco.edu.co To whom correspondence may be addressed
Many well pressure data coming from long and narrow reservoirs which result from either fluvial deposition of faulting cannot be completely interpreted by conventional analysis since some flow regimes are not conventionally recognized yet in the oil literature. This narrow geometry allows for the simultaneous development of two linear flow regimes coming from each one of the lateral sides of the system towards the well. This has been called dual linear flow regime. If the well is off-centered with regards to the two lateral boundaries, then, one of the linear flow regimes vanishes and, then, two possibilities can be presented. Firstly, if the closer lateral boundary is close to flow the unique linear flow persists along the longer lateral boundary. It has been called single linear flow. Following this, either steady or pseudosteady states will develop. Secondly, if a constant-pressure closer lateral boundary is dealt with, then parabolic flow develops along the longer lateral boundary. Steady state has to be developed once the disturbance reaches the farther boundary.
This study presents new equations for conventional analysis for the dual linear, linear and parabolic flow regimes recently introduced to the oil literature. The equations were validated by applying them to field and simulated examples.
Keywords: reservoir, linear flow, radial flow, parabolic flow.
Muchos datos de presión procedentes de yacimientos alargados y angostos, resultado de depósitos fluviales o callamiento, no pueden interpretarse por métodos convencionales puesto que existen algunos regímenes de flujo desconocidos en la literatura referente al método convencional. Esta geometría estrecha del yacimiento permite el desarrollo simultáneo de dos regimenes de flujo lineales, actuando a ambos lados alargados del yacimiento y dirigiéndose al pozo. Éste ha sido llamado flujo dual lineal. Cuando el pozo está descentrado con respecto a una de las dos fronteras laterales, uno de los flujos lineales desaparece y pueden presentarse dos posibilidades: primero, si la frontera más cercana es cerrada, un único flujo lineal persiste a lo largo de la prueba. Éste ha sido llamado flujo lineal único. Después de éste, se desarrollará el estado pseudoestable o estable. Sforndo, si la frontera cercana es de presión constante, entonces se desarrolla el flujo parabólico hacia el lado más largo del yacimiento. El estado estable deberá desarrollarse, una vez la perturbación haya alcanzado la frontera más lejana .
Este estudio presenta ecuaciones nuevas para análisis convencional para los regímenes de flujo dual lineal, lineal único y parabólico recientemente introducidos a la literatura petrolera. Las ecuaciones fueron validadas mediante su aplicación a ejemplos simulados y de campo.
Palabras clave: yacimientos, flujo lineal, flujo radial, flujo parabólico.


INTRODUCTION
Very few researchers have focused their attention on long and narrow systems such as channel sands (Figure 1.a). Among them, we can name Ehlig-Economides and Economides (1985), Massonet, Norris, and Chalmette (1993), Mattar (1997), Nutakki and Mattar (1982) and Wong, Mothersele, Harrington, and Cinco-Ley (1986). A better description of channelized systems and a detailed interpretation technique was presented by Escobar, Saavedra, Hernández, C.M., Hernández, Y.A., Pilataxi, and Pinto (2004) who classified the linear flow regime into two closely related names: “dual linear flow” and “single linear flow” regimes as sketched in Figure 1.b. When the well is off-centered with respect to the lateral sides, firstly, we observe two linear flow regimes coming from both lateral sides of the well. They called this “dual linear flow”. Once the close-to-flow closer boundary is reached by the transient wave, a unique linear flow remains throughout the longer lateral boundary. Single linear flow can also be present when the well is located very close to one lateral boundary as shown in Figure 1.c. Escobar et al. (2004) called this “single linear flow” or simply, “linear flow”. However, if the closer boundary is at constant pressure, then “parabolic flow” develops and steady state follows this flow regime. The parabolic flow is recognized on the pressure derivative curve by a negative 0,5-slope straight line. The reader is referred to Escobar, Muñoz, Sepúlveda, Montealegre, and Hernández (2005a) and Escobar, Muñoz, Sepúlveda, J.A., and Montealegre (2005b) for a detailed explanation of the parabolic flow regime.
MATHEMATICAL FORMULATION
The dimensionless time quantities used by Escobar et al. (2004) were:
Dimensionless reservoir width and well position, as sketched in Figure 1.a, Escobar et al. (2004), are:
Dimensionless pressure is defined by Earlougher (1977) as:
Nutakki and Mattar (1982) presented an equation for the linear flow, as follows:
Escobar et al. (2004) and Escobar, Hernández, Y.A., and Hernández, C.M. (2007) have described the differences between the dual linear flow and the single linear flow occurring in elongated systems. Escobar et al. (2004) found out that Equation 8 was mistaken, so they proposed a new equation to describe dual linear, and single linear as well, flow regimes, such as:
Dual linear flow analysis
As depicted in Figure 1.b, this type of flow is formed by to linear flows occurring from each lateral side of the reservoir towards the well, so that they have an opposite direction to each other.
Replacing Equations 1 through Equation 7, into Equation 9 will yield:
For pressure buildup analysis, application of time superposition is required, therefore Equation 9 becomes:
Which implies that a cartesian plot of ∆P vs. either or will yield a straight line during dual linear flow behavior which slope, mDLF, and intercept, bDLF, are used to obtain reservoir width, YE, and dual linear skin factor, sDL.
Notice that Equation 13 is different to Equation 14 presented by Wong et al. (1986). However, our mathematical model agrees with those presented by Nutakki and Mattar (1982) and Ehlig-Economides and Economides (1985).
Single linear flow analysis
Regarding Figure 1.b, once the pressure disturbance reaches the first lateral boundary, one of the linear flow no longer exists, then a unique linear flow prevails which is called single linear flow regime. This flow also can take place when the well is very close to one of the lateral boundaries as sketched in Figure 1.c.
Replacement of Equation 1 through Equation 7 into Equation 10 will yield:
For pressure buildup analisis:
Equation 15.a and Equation 15.b indicates that a plot of ∆P vs. either or in cartesian coordinates will yield a straight line during linear flow behavior which slope, mLF, and intercept, bLF, are used to obtain reservoir width, YE, and linear skin factor, sL, respectively:
Parabolic flow analysis
The reader is referred to Escobar et al. (2005) for a better description of this type of flow. Escobar et al. (2004), Escobar et al. (2005a) and Escobar et al. (2005b) introduced the governing equation for pressure behavior dominated by parabolic flow, as follows:
Replacing of Equation 1 through Equation 7 into Equation 18 will yield:
For buildup analysis, superposition is required; then, the pressure equation is:
A cartesian plot of ∆P vs. either 1/ or will yield a straight line during the parabolic dominated region which slope, mPB, and intercept, bPB, are used to obtain well position, bx, and parabolic skin factor, sPB, respectively, as:
Sui, Mou, Bi, Den, and Ehlig-Economides (2007) have also found the behavior depicted by a negative half-slope once linear flow regime has vanished in elongated reservoirs. However, they called it “dipolar flow”.
NUMERICAL EXAMPLES
Simulated example
The synthetic pressure drawdown reported in Figure 2 was performed for a well inside a rectangular reservoir using the data given in the second column of Table 1.
The slope and intercept (mDLF = 1077 psi/h0,5, bDLF = 2792 psi) obtained from Figure 3 were applied into Equations 12 and Equation13 to yield an estimation of YE = 249,6 ft and sDL = 4,9, respectively. Also, from Figure 4 values of mLF = 1968,4 psi/h0,5 and bDLF = 4921 psi were read to be applied into Equation 16 to estimate a YE value of 242 ft and Equation 18 was used to estimate a sL of -8,6. A summary of the results are given in Table 2 . We observe a very good agreement between the calculated and the input YE value.
Field example
Escobar et al. (2004) presented an example of a pressure drawdown test run in a well in a channelized reservoir in the Eastern Planes basin in Colombia. Reservoir and well parameters are given in the third column of Table 1 and pressure and pressure derivative data are given in Figure 5.
As shown in Figure 5, radial, dual linear, parabolic flows and steady state are observed. Escobar et al. (2004) reported a permeability of 441 md and a mechanical skin factor of -4,9 determined using the TDS technique, Tiab (1993). As shown in Figure 6, the semilog slope is found to be 140 psi/cycle and P1h = 1158 psi. The conventional equation to estimate the mechanical skin factor is given by Earlougher (1977):
Using Equation 24 the mechanical skin factor, s, is -4,6. From Figure 7, the slope during dual linear flow regime was 150,82 psi/h0,5 and an intercept value of 19,4 psi was also determined. A reservoir width, YE of 350 ft was determined using Equation 12. The dual linear skin factor, sDL, estimated with Equation 13 was 0,17.
From Figure 8, the slope during parabolic flow was determined to be -851,6 psi*h0,5 and the intercept was 731 psi. The well position along the x-direction, bx, was estimated with Equation 21 to be 277 ft and the parabolic skin factor found from Equation 22 was 6,1. The following results were obtained by simulation using a commercial and well-known well test interpretation software:
k = 416 md
s = -5,1
Well distance to the north = 85 ft
Well distance to the constant-pressure
boundary = 278 ft
Well distance to the no-flow boundary = 343 ft
Well distance to the south = 421 ft
From the above data YE = 85 + 421 = 506 ft, bx = 2785 ft and XE = 279 + 343 = 621 ft. Escobar et al. (2004) did not report the parabolic skin factor, however, it will be estimated using their Equation 9, as follows:
The following information was read from the pressure and pressure derivative plot shown in Figure 4:
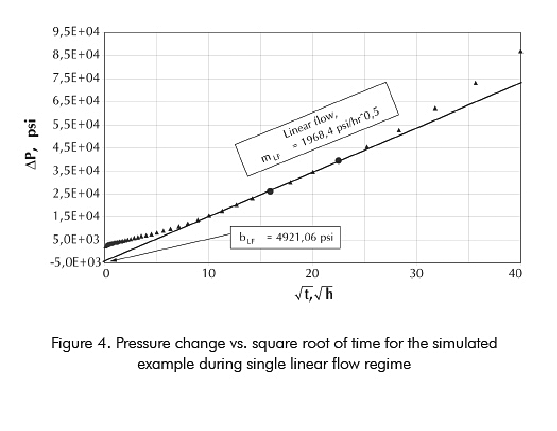
tPB = 10,157 h
(t*∆P’)PB = 132,873 psi
∆PPB = 458,466 psi
Using bx = 277, k = 441 md and YE = 350 ft a sPB = 6,1 was calculated from Equation 25. Table 3 summarizes the results for this example. Notice there that the only unmatched value is reservoir witdh obtained from simulation. The remaining estimations are in close agreement. Needless to say, how well the estimated total skin factor agrees with the one obtained from the TDS technique.
ANALYSIS OF RESULTS
As expected, reliable results (Table 3) were obtained from the application of the developed set of equations to the worked examples. For the numerical example we observe that the reservoir width value, YE, obtained from Equation 12 agrees almost perfectly with the value used to run the simulation. The value of reservoir width from Equation 16 has a relative small error of 3,2 % compared to the original value. We can state that there is a good agreement between the two values. No discussion can be established regarding the kin factor since there is no point of comparison; commercial simulators use space superposition to generate the pressure curves.
As far as the field problem is concerned, we observe a good matching between the values obtained from the equations presented in this study and those results obtained from both TDS technique and commercial software. See Table 3. The value of reservoir width obtained from numerical simulation (commercial software) does not agree well with the results of this study. However, the matching of the simulated and field data were not the best, therefore, the difference may be due to it.
CONCLUSIONS
- New relationships to characterize the single linear flow regime for the conventional method of well test interpretation have been presented for estimation of reservoir width and linear skin factor. Also, equations for conventional analysis during parabolic flow regime for the determination of the well position along the x-direction and the parabolic skin factor are presented. The equations were tested and verified by their application to numerical examples and compared to results obtained from simulation and the TDS technique.
- Since most of simulators use superposition (the image method) for estimating the pressure behavior of a well inside a rectangular system, they do not estimate neither linear, nor dual, nor parabolic skin factors, therefore, comparison of those parameters was referred to the TDS technique.
- Even though, the traditional conventional analysis is a very powerful and respected tool, the TDS technique can provide, in a much more practical way, more parameters from the same test.
ACKNOWLEDGMENTS
The authors gratefully acknowledge the financial support of Universidad Surcolombiana (Neiva-Huila, Colombia) for the development of this work.
REFERENCES
Earlougher, R.C., Jr. (1977). Advances in well test analysis. Monograph Series 5, SPE, Dallas, TX. [ Links ]
Ehlig-Economides, C., & Economides, M.J, (1985). Pressure transient analysis in an elongated linear flow systems. J. Petroleum Scien. and Engineer. December, 25: 839-847. [ Links ]
Escobar, F.H., Saavedra, N.F., Hernández, C.M., Hernández, Y.A., Pilataxi, J.F., & Pinto, D.A. (2004). Pressure and pressure derivative analysis for linear homogeneous reservoirs without using type-curve matchin. 28th Annual SPE International Technical Conference and Exhibition, Abuja, Nigeria, Aug. 2-4. SPE 88874. [ Links ]
Escobar, F.H., Hernández, Y.A., & Hernández, C.M. (2007). Pressure transient analysis for long homogeneous reservoirs using TDS technique. J. Petroleum Scien. and Engineer., 58: 68-82. [ Links ]
Escobar, F.H., Muñoz, O.F., Sepúlveda, J.A., Montealegre-M., M., & Hernández, Y.A. (2005a). Parabolic flow: a new flow regime found in long, narrow reservoirs. XI Congreso Colombiano del Petróleo, Bogotá, Colombia, Oct. 18-21. [ Links ]
Escobar, F.H., Muñoz, O.F., Sepúlveda, J.A., y Montealegre, M. (2005b). New finding on pressure response in long, narrow reservoirs .CTYF - Ciencia, Tecnología y Futuro.3(1), 151-160 [ Links ]
Massonet, G.J., Norris, R.J., & Chalmette, J-C. (1993). Well test interpretation in geologically complex channelized reservoirs. 68th Annual Technical Conference and Exhibition of the Society of Petroleum Engineers, Houston, TX. Oct. 3-6. SPE 26464. [ Links ]
Mattar, L. (1997). Derivative analysis without type curves. The Petroleum Society. 48th Annual Technical Meeting of the Petroleum Society, Calgary, Alberta, Canada, June 8-11. SPE 97-51. [ Links ]
Sui, W., Mou, J., Bi, L., Den, J., y Ehlig-Economides, C. (2007). New Flow Regimes for Well Near-Constant-Pressure Boundary. SPE Latin American and Caribbean Petroleum Engineering Conference. Buenos Aires, Argentina. April 15-18. SPE 106922. [ Links ]
Nutakki, R. And Mattar, L. (1982). Pressure transient analysis of wells in very long narrow reservoirs. 57th Annual Fall Technical Conference and Exhibition of the Society of Petroleum Engineers, Dallas, TX, Sept. 26-29. SPE 11221. [ Links ]
Tiab, D. (1993). Analysis of pressure and pressure derivative without type-curve matching: 1- skin factor and wellbore storage. SPE Production Operations Symposium, Oklahoma City, OK. March 21-23, SPE 25423, 203-216. Also, J. Petroleum Scien. and Engineer., (1995), 12 (3): 171-181. [ Links ]
Wong, D.W., Mothersele, C.D., Harrington, A.G., & Cinco-Ley, H. (1986). Pressure transient analysis in finite linear reservoirs using derivative and conventional techniques: field examples. 61st Annual technical Conference and Exhibition of the Society of Petroleum Engineers, New Orleans, LA, Oct. 5-8, SPE 15421. [ Links ]
(Received May 30, 2006; Accepted Sept. 24, 2007)










































